Mastering Financial Calculations: Perpetuities and Annuities Simplified

Understanding Perpetuities: A Financial Formula for Indefinite Cash Flows

When dealing with financial calculations, perpetuities are a key concept as they represent assets that provide a fixed sum each year without an end. These cash flows are consistent and occur at regular intervals. The formula for calculating the return on a perpetual cash flow is straightforward: you divide the periodic cash flow by the discount rate. This method allows investors to determine the present value of a cash flow expected to continue indefinitely.

Calculating Present Value of Perpetual Cash Flows

To calculate the present value of perpetual cash flows, use the formula:

Present Value = Periodic Cash Flow / Discount Rate

For example, if you have a $1 billion annual cash flow with a perpetual discount rate of 10%, the present value is $10 billion.

Adjusting for Future-Starting Perpetuities

If a perpetuity doesn't start immediately but at a future date, the calculation adjusts to include a present value factor. This factor discounts the future value back to the present, taking into account the number of periods until the perpetuity begins. The adjusted formula involves multiplying the standard perpetuity present value by the present value factor (1 / (1 + interest rate)^n), where n is the number of periods until the start.

Annuities: Fixed Cash Flows Over a Specific Period

Annuities are similar to perpetuities but they occur over a finite number of periods. The main characteristics of annuities are equal cash flows at consistent intervals. The present value of an annuity can be calculated using the formula:

Present Value Annuity Factor = Periodic Cash Flow x (1 / interest rate - 1 / (interest rate x (1 + interest rate)^n))

By applying this formula, you can easily determine the present value of cash flows that would otherwise be laborious to calculate individually over many periods.

Real-World Application: Lottery Winnings

Consider the advertised jackpot of a lottery paid in installments over 30 years. Using the annuity formula, you can calculate the true present value of the prize rather than simply summing up the yearly payments.

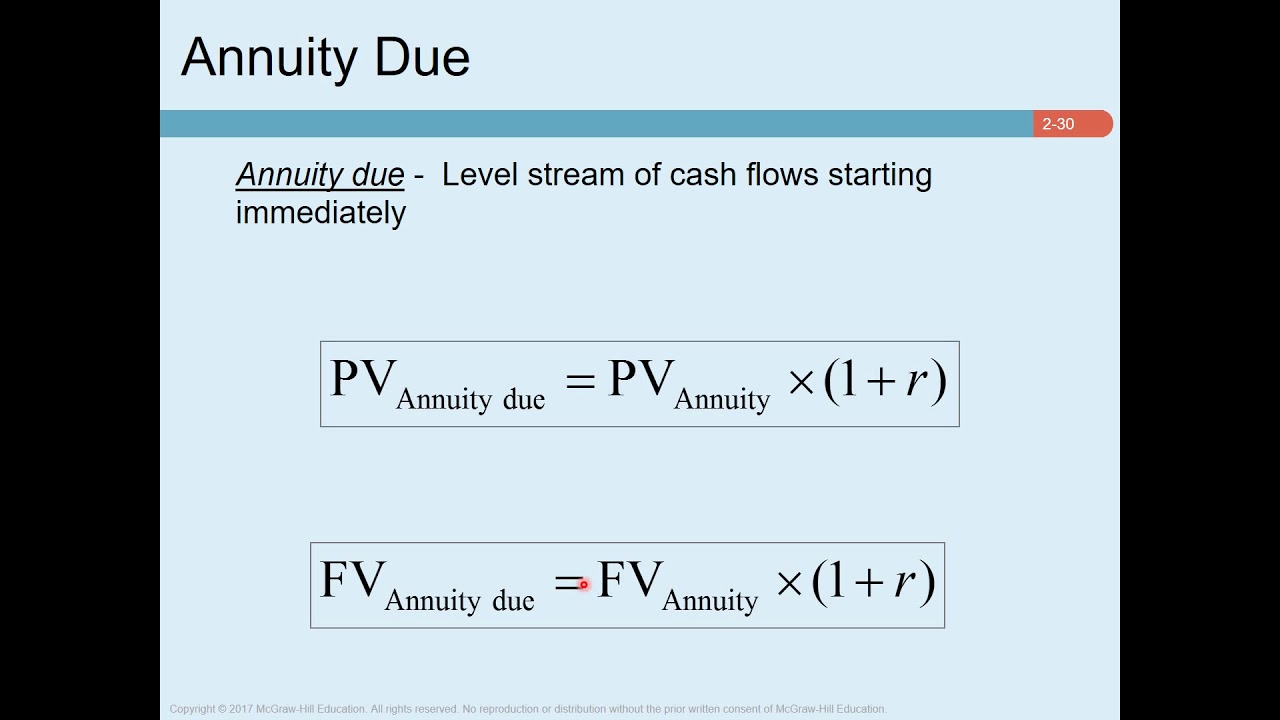
Types of Annuities: Ordinary vs. Annuity Due

Annuities can be classified as either ordinary annuities or annuities due. Ordinary annuities begin at the end of the first period, while annuities due start at the beginning. The calculation for an annuity due adds an extra factor to the formula for an ordinary annuity, accounting for the immediate start of payments.

Formula Adjustments for Annuity Due

To calculate the present value of an annuity due, you simply multiply the ordinary annuity formula by (1 + interest rate). Likewise, the future value of an annuity due multiplies the future value annuity formula by the same term.

Utilizing Annuities: Paying Off Loans

Annuities are not just for investments; they also apply to loan payments. For instance, if you're financing a purchase with installments, you can use the annuity formula to determine the size of each payment based on the present value of the loan.

Future Value of Annuities: Looking Ahead

The future value of an annuity formula helps predict the value of a series of cash flows at a future date. It's especially useful for long-term investments where you want to know the worth of consistent payments after several years.

Growing Perpetuities: When Cash Flows Increase Over Time

A growing perpetuity is a variation where the cash flow increases at a constant rate each period. The present value of a growing perpetuity incorporates the growth rate into the formula:

Present Value = Periodic Cash Flow / (Discount Rate - Growth Rate)

This adjustment shows how growth impacts the present value of cash flows over an indefinite period.

In conclusion, understanding and applying the formulas for perpetuities and annuities can significantly simplify financial calculations, particularly when dealing with complex or extended cash flows. Whether you're calculating lottery winnings, loan payments, or the future value of investments, these financial shortcuts can streamline the process and provide valuable insights into the worth of financial assets.

For a more detailed explanation and examples, watch the full lecture on YouTube.