Create articles from any YouTube video or use our API to get YouTube transcriptions
Start for freeThe Nuances of Interest Rates: Effective vs. Annual Percentage Rates
When it comes to investments and calculating returns, understanding the distinction between effective interest rates and annual percentage rates (APRs) is crucial. These concepts are fundamental for investors navigating the world of bonds and other interest-bearing financial instruments.
Annual Cash Flows vs. Semi-Annual Interest Payments
In some countries, such as France and Germany, government bonds pay out interest annually. This means that investors receive their interest payments once per year. However, this is not a universal standard. For example, in the United States and the United Kingdom, government bonds typically pay interest semi-annually.
How Semi-Annual Compounding Affects Returns
When a U.S. government bond is quoted with a 10% interest rate, this does not mean the investor receives the full 10% at the end of the year. Instead, the investor receives 5% after the first six months and another 5% after the second six months. This semi-annual payment structure allows investors to potentially earn additional interest on the first payment over the second six-month period.
Example of Semi-Annually Compounded Interest:
- Invest $100 in a bond at 10% interest compounded semi-annually.
- After the first six months, your investment grows by 5%, becoming $105.
- By the end of the year, your initial investment becomes $110.25 after the second 5% increase.
This example illustrates that a 10% interest rate compounded semi-annually effectively yields a 10.25% interest rate when compounded annually.
Effective Interest Rate vs. Annual Percentage Rate
Effective Interest Rate:
The effective interest rate annualizes the interest rate using the principle of compound interest. It considers the impact of earning interest on previously earned interest, leading to higher returns than the nominal rate.
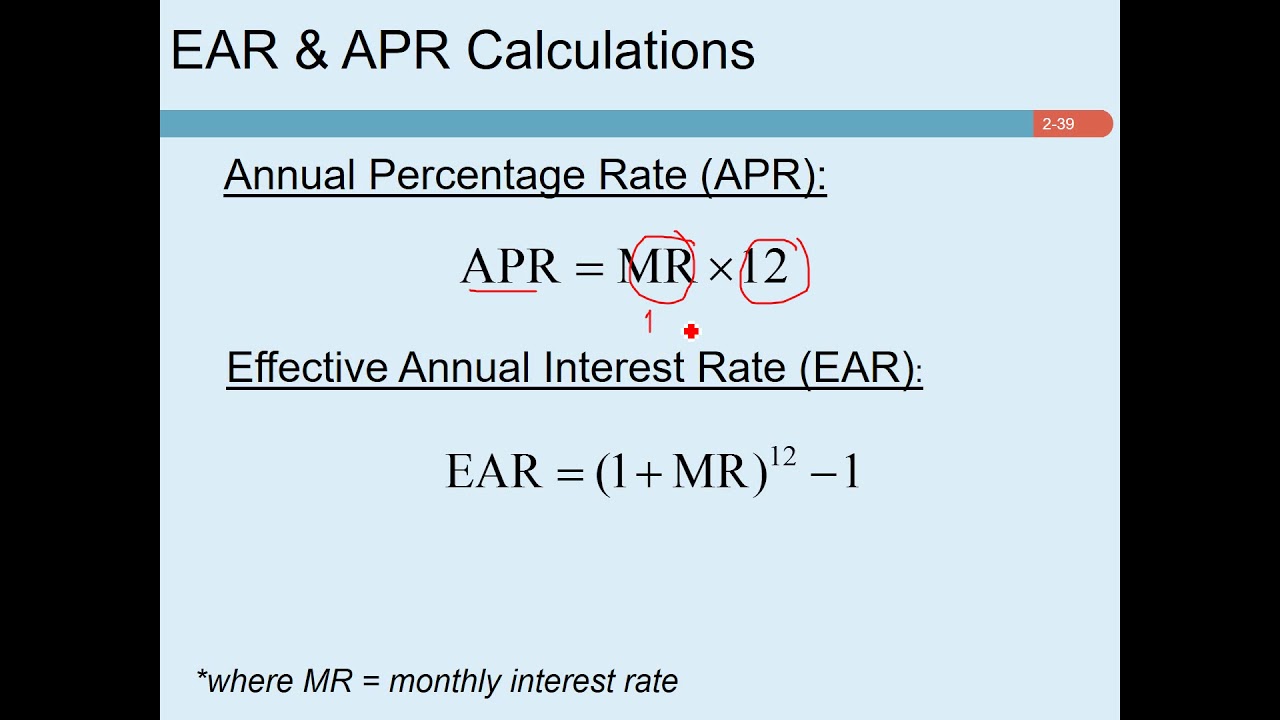
Annual Percentage Rate (APR):
The APR, on the other hand, is annualized using simple interest. It does not take into account the compounding of interest within the year. The APR is calculated by multiplying the periodic interest rate by the number of periods in a year.
Clarifying with an Example:
If you receive a monthly interest of 1%, the APR would be:
- Monthly interest: 1%
- Number of months: 12
- APR: 1% * 12 = 12%
The APR does not consider the compounding effect – that interest can be earned on interest. Conversely, the effective annual rate for a 1% monthly interest rate would be higher than 12% because each month's interest would earn additional interest throughout the year.
Calculating Effective Annual Rate
Given a monthly interest rate of 1%, the effective annual rate takes into account the interest earned on interest, resulting in a rate higher than the APR. For a 1% monthly rate, the effective annual rate would be approximately 12.68%, reflecting the compounding effect.
Conclusion
The distinction between effective interest rates and APRs is significant for investors who want to accurately project their investment growth. Understanding these concepts ensures better financial decision-making and clearer expectations of investment returns.
For a detailed discussion on simple and effective interest rates and to see these concepts in action, watch the original video here.
Thank you for joining us in this exploration of interest rates, and stay tuned for more financial insights.


