Create articles from any YouTube video or use our API to get YouTube transcriptions
Start for freeUnderstanding the Efficient Frontier with Multiple Assets
Investing is an art and a science, with risk and return at the heart of every decision. The concept of the Efficient Frontier plays a crucial role in helping investors understand the best possible investment combinations. In our previous discussion, we delved into the Efficient Frontier for two assets, but today's focus expands to a more complex scenario involving multiple assets.
What Is the Efficient Frontier?
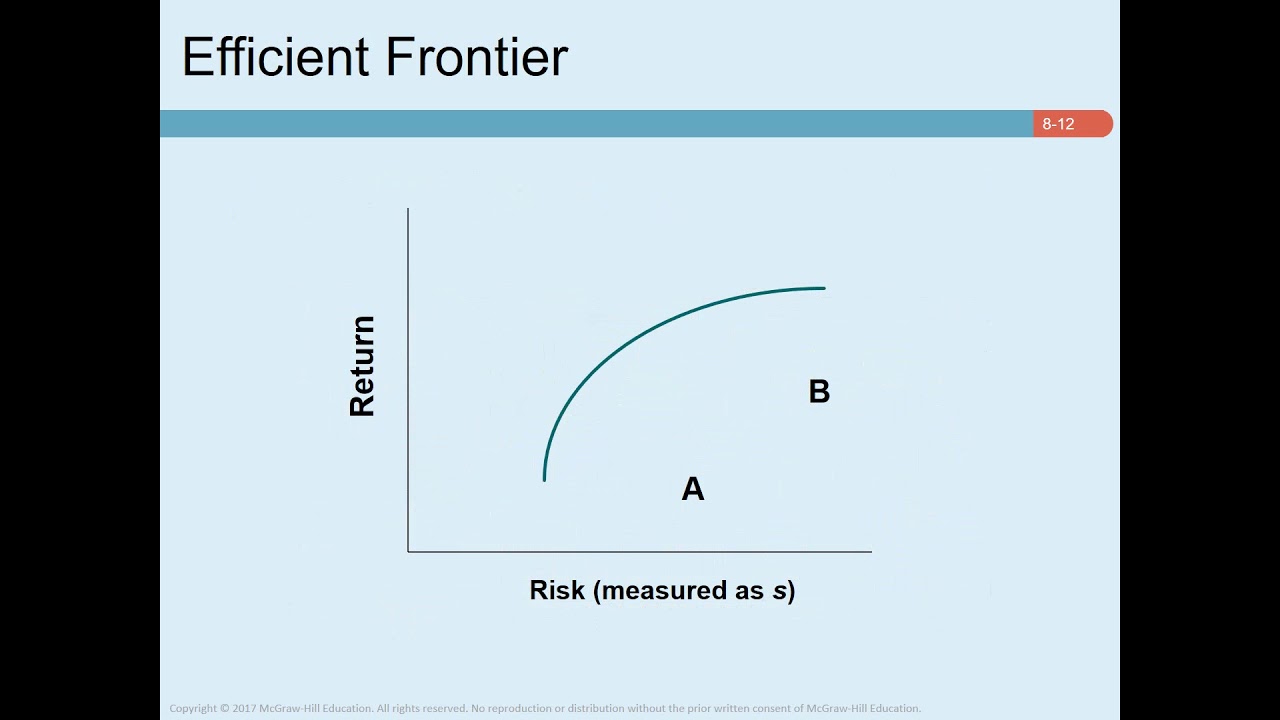
The Efficient Frontier is the set of optimal portfolios that offer the highest expected return for a defined level of risk. This frontier is represented as a curve on a graph where the x-axis denotes risk (measured by standard deviation) and the y-axis represents expected return.
Building a Multi-Asset Portfolio
When constructing a portfolio, investors aren't confined to a pair of assets. Let's say you're considering a selection from ten different stocks. By analyzing each company's prospectus and historical data, forecasts of returns can be determined. For instance, if 'Company X' showcases the highest expected return, it becomes a prime candidate for your portfolio, whereas a company like 'Campbell Soup,' with its lower return, might be approached with caution.
Risk, Return, and Correlation
Investors use past performance, typically spanning five to ten years, to gauge the risk of each stock and the correlation between pairs. On a graph, each diamond represents the risk-return combination of an individual security. By holding various proportions of these ten securities, you can achieve a wide array of risk and return combinations.
The Goal of Investment Strategies
The objective is to move towards the left of the graph (lower risk) and upwards (higher return). Ideally, any point along the red line, indicating the Efficient Frontier, represents an efficient portfolio. These portfolios are 'efficient' because they provide the highest expected return for a given level of risk.
Risk Appetite and Portfolio Selection
Your choice of where to invest along the Efficient Frontier is guided by your risk tolerance. If you're risk-averse, you'll opt for lower risk and return. Conversely, if you're willing to accept more risk, you'll target a higher return.
The Impact of Borrowing and Lending
In the real world, investors can also borrow and lend money, often at a risk-free rate. This introduces the concept of the Optimal Risky Portfolio, which is the touchpoint between the Efficient Frontier and the Security Market Line that we'll discuss in an upcoming video.
Diversifying with Different Asset Classes
The Efficient Frontier isn't static. If you introduce other asset classes like currencies, real estate, or bonds into your portfolio, your frontier shifts leftward. This means for the same risk level, you can achieve a higher return or maintain the same return with reduced risk. Diversification reduces risk because different asset classes often have lower correlations with each other.
Measuring Portfolio Performance with Sharpe Ratio
To assess how well your portfolio performs on a risk-adjusted basis, the Sharpe Ratio is used. It is calculated as the difference between the portfolio's return and the risk-free rate, divided by the portfolio's standard deviation. A higher Sharpe Ratio signifies a more favorable risk-adjusted return.
Making the Right Investment Choice
Your position on the Efficient Frontier should align with your risk appetite, whether you're seeking high-risk-high-return or low-risk-low-return investments.
In conclusion, understanding the Efficient Frontier in the context of multi-asset portfolios is vital for making informed investment decisions. It allows investors to identify the most efficient combination of assets that match their risk tolerance and return objectives. As we continue to explore investment strategies, remember that the Efficient Frontier is a powerful tool for optimizing your portfolio's performance.
For a more visual and detailed explanation, watch the original video here.


