Create articles from any YouTube video or use our API to get YouTube transcriptions
Start for freeUnderstanding Present and Future Values in Finance
Welcome to the second week of our financial lecture series where we delve into one of the most fundamental concepts in finance: the time value of money. This principle states that a dollar today holds more value than a dollar tomorrow due to its potential earning capacity. This week, we will explore how to calculate present and future values, and why these calculations are crucial for investors and corporations alike.
The Time Value of Money
Last week, we discussed the time value of money and established that immediate cash flows are more valuable because they can be invested to earn returns. In this chapter, we'll build on that knowledge by learning the mechanics behind calculating present and future values.
Calculating Present Values
Present value is the current worth of a future cash flow. For instance, if you're to receive $100 at the end of the year, the present value is what that $100 is worth today. To calculate present value, we need to understand the concepts of compounding and discounting, and the differences between nominal and effective interest rates.
The Mechanics of Future Value
Future value, on the other hand, is what a current amount of money will be worth in the future given a specific rate of return. The formula to calculate future value is:
Future Value = Current Cash Flow x (1 + Interest Rate)^Time Period
Let's illustrate this with an example. If you invest $100 at a 7% annual interest rate for two years, the future value is calculated by multiplying $100 by (1 + 0.07)^2.
Compound Interest vs. Simple Interest
There are two types of interest: simple and compound. Simple interest is earned only on the principal amount, while compound interest is earned on both the principal and the accrued interest. This difference can significantly affect how investments grow over time.
Graphical Illustration of Investment Growth
A graph can show how money grows at different interest rates. A zero percent rate keeps the money static, while higher rates accelerate growth. This visual representation emphasizes the impact of interest rates on investment growth.
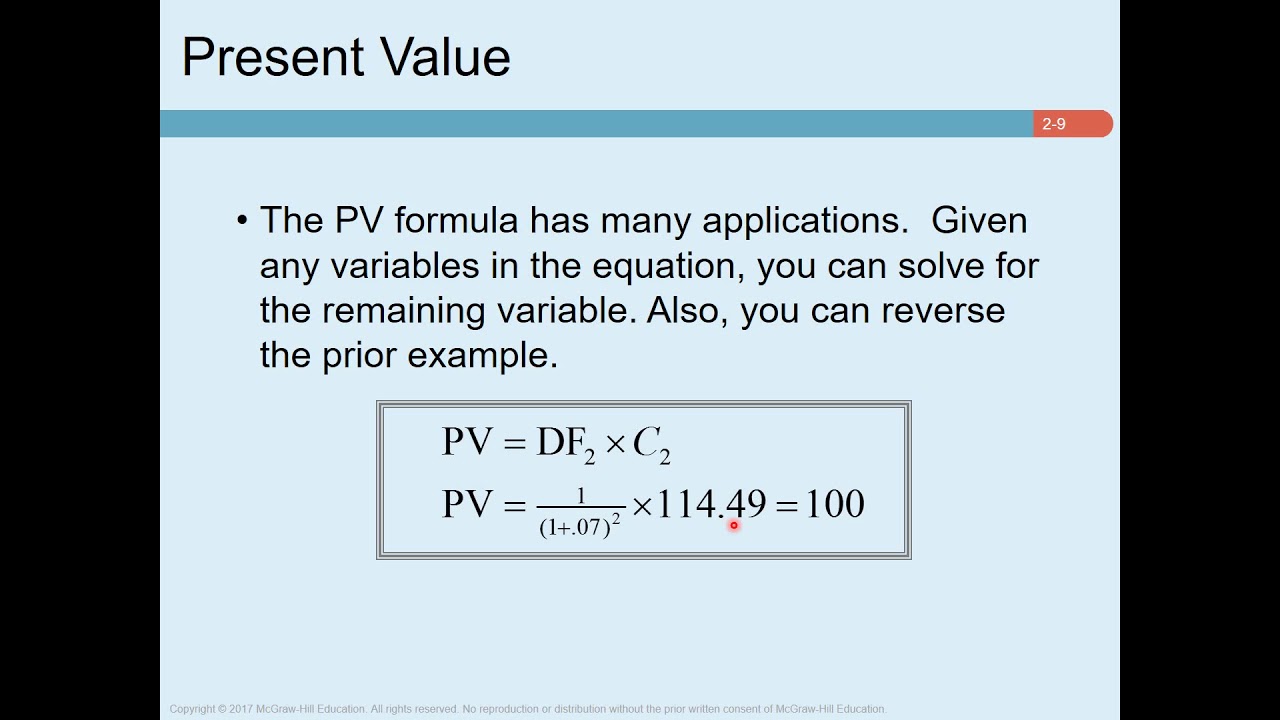
Discounting to Present Value
To calculate the present value, we use a discount factor, which is the present worth of $1 expected in the future at a specific interest rate. The formula for the discount factor is:
Discount Factor = $1 / (1 + Interest Rate)^Time Period
Applying this to our earlier example, if we expect to receive $114.49 at a 7% interest rate in two years, the present value would be calculated by multiplying the discount factor by the expected cash flow.
Deciding on Investment Opportunities
The net present value (NPV) rule helps determine whether an investment is worth pursuing. If the present value of cash inflows exceeds the initial investment, the NPV is positive, and the investment adds value to the company, thus should be accepted.
In our example of constructing an office building, if the cost is $700,000 and the expected sale price is $800,000 after one year, with a 7% cost of capital, we calculate the present value of the expected sale price and compare it to the investment cost. A positive NPV indicates a worthwhile investment.
Conclusion
This week's lesson sets the foundation for making informed capital investments by understanding the value of money over time. Stay tuned for additional topics and deepen your financial acumen by following the series. To ensure you've got a grasp on these concepts, don't forget to check out the next videos for more insights.
For a detailed walkthrough of these calculations, watch the original video here.


