Mastering Integration: The Inverse Operation of Differentiation Explained

Introduction to Integration and Differentiation

Integration stands as the antithesis to differentiation in the realm of calculus. Understanding differentiation, wherein we multiply by the power and then subtract one from the power, lays the groundwork for grasping integration. This article delves into the fundamentals of integration, reversing the process of differentiation to solve various mathematical problems.

Understanding Differentiation

Differentiation involves a straightforward procedure:

• Multiply by the power of the variable.
• Subtract one from the power.

For instance, differentiating $3x^2$ results in $6x$, following the rule of multiplying by the power (2 in this case) and then reducing the power by one.

The Process of Integration

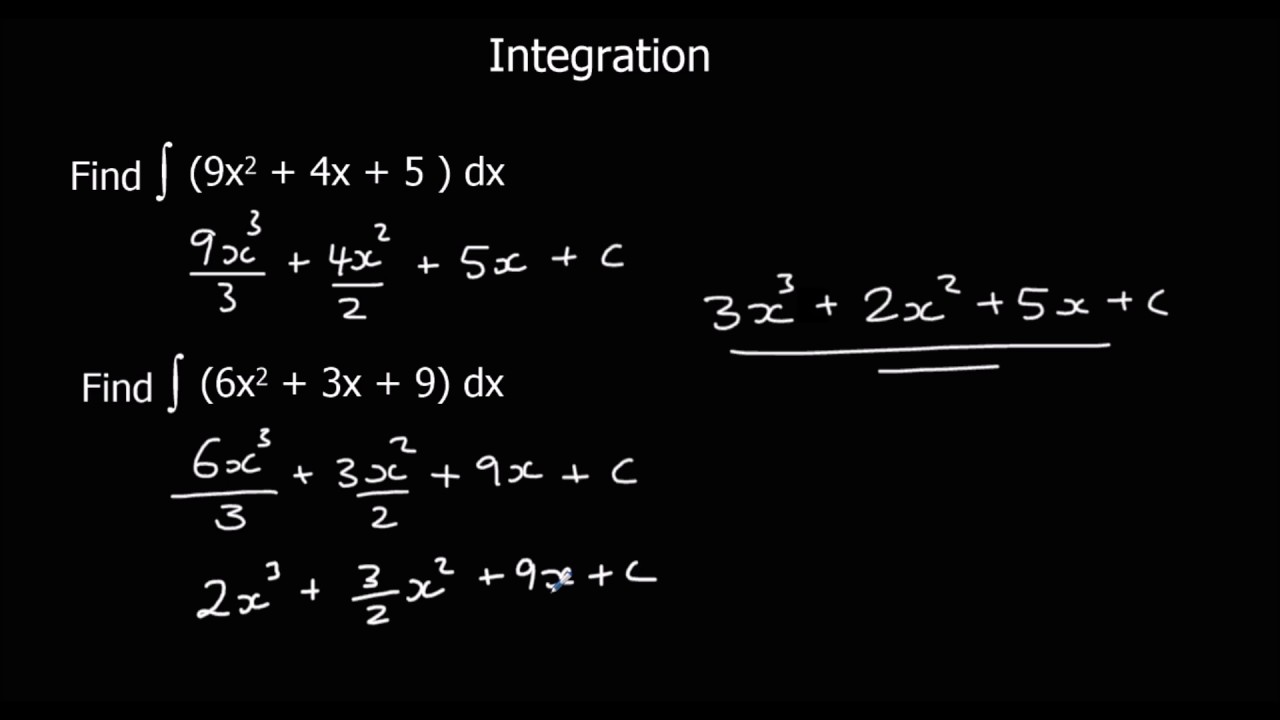
Integration reverses the steps taken in differentiation. Instead of subtracting one from the power, we add one, and instead of multiplying, we divide by the newly obtained power. An integral part of integration is the addition of a constant, denoted as $C$, to account for any constant term that differentiation might erase.

Example of Integration

Given the differentiation result $6x^2 + 18x + 5$, to integrate:

1. Add one to the power of each term.
2. Divide by the new power.
3. Add the constant $C$ to represent any constant term lost during differentiation.

Thus, integrating $6x^2 + 18x + 5$ yields $2x^3 + 9x^2 + 5x + C$.

Exercises in Integration

The practice of integration is best illustrated through examples. One exercise involves integrating $x^2 + 4x + 10$, applying the step-by-step process of adding one to the power, dividing by the new power, and appending $C$. The result is a simplified expression that represents the antiderivative of the given function.

Tackling More Complex Integrals

Some integrals require preliminary simplification, such as converting $10/x^2$ to $10x^{-2}$ before applying the integration steps. Similarly, expressions involving roots, like $\ ext{sqrt}(x)$, are rewritten in power form, e.g., $x^{1/2}$, to facilitate integration.

Practical Application: Finding an Expression for $y$ in Terms of $x$

Integration proves invaluable in deriving expressions for $y$ based on the rate of change, $dy/dx$. Given $dy/dx = 3x^2 + 5x + 10$ and an initial condition (e.g., $x=2$, $y=3$), integration allows us to find an explicit formula for $y$ in terms of $x$, incorporating the determined constant $C$.

Conclusion

Integration, the inverse of differentiation, is a cornerstone of calculus that enables the determination of antiderivatives and the solving of complex mathematical problems. Through the methodical addition of one to the power, division by the new power, and the inclusion of $C$, integration unravels the mysteries hidden within differential equations. Practice with a variety of functions sharpens understanding and proficiency in applying this essential mathematical operation.

Explore more about integration and its applications by watching the original video here.