Decoding Risk and Reward: Understanding NPV and Investment Decisions

Understanding the Relationship Between Risk and Return

In the world of finance, the balance between risk and reward is fundamental. Investors constantly evaluate the potential returns of an investment against the inherent risks involved. One of the key concepts in this evaluation is the Net Present Value (NPV), a financial metric that helps determine the profitability of an investment.

The Basics of NPV and Investment Decisions

Net Present Value is a critical concept for anyone involved in making investment decisions. It represents the difference between the present value of cash inflows and the cost of investment (outflows). A positive NPV means that the present value of the expected cash inflows from an investment exceeds the initial cost, indicating a profitable opportunity.

For instance, consider an office building project with a construction cost of $700,000. If the expected selling price at the end of the first year is $800,000 and the cost of funds is 7%, discounting the future cash inflow gives us a present value of $747,664. Since the present value is greater than the initial investment, the project would be accepted based on the NPV rule.

Risk Adjustments in Discount Rates

However, financial investments are never without risk. The $800,000 expected return is not guaranteed, and a savvy investor would compare the risk of the office building project with a risk-free investment like government securities. If government securities offer a 7% return with no risk, any riskier investment would necessitate a higher return to be attractive.

This leads to the principle that a safe dollar is worth more than a risky dollar. To account for the increased risk in the office building project, the cash inflows should be discounted at a higher rate, reflecting the required additional return. For example, if the risk-adjusted discount rate is 12%, the present value of the expected cash inflows drops to $714,286. Despite the higher discount rate, if the NPV remains positive, the project may still be worth pursuing.

The Correlation Between Risk and Discount Rates

Investments come with varying levels of risk, which directly influences the discount rates applied to their expected cash flows. A high-risk project will have a higher discount rate, while a low-risk project will have a lower discount rate. This differentiation is vital for investors seeking to maximize returns while managing risk exposure.

The NPV Rule and Investment Acceptance

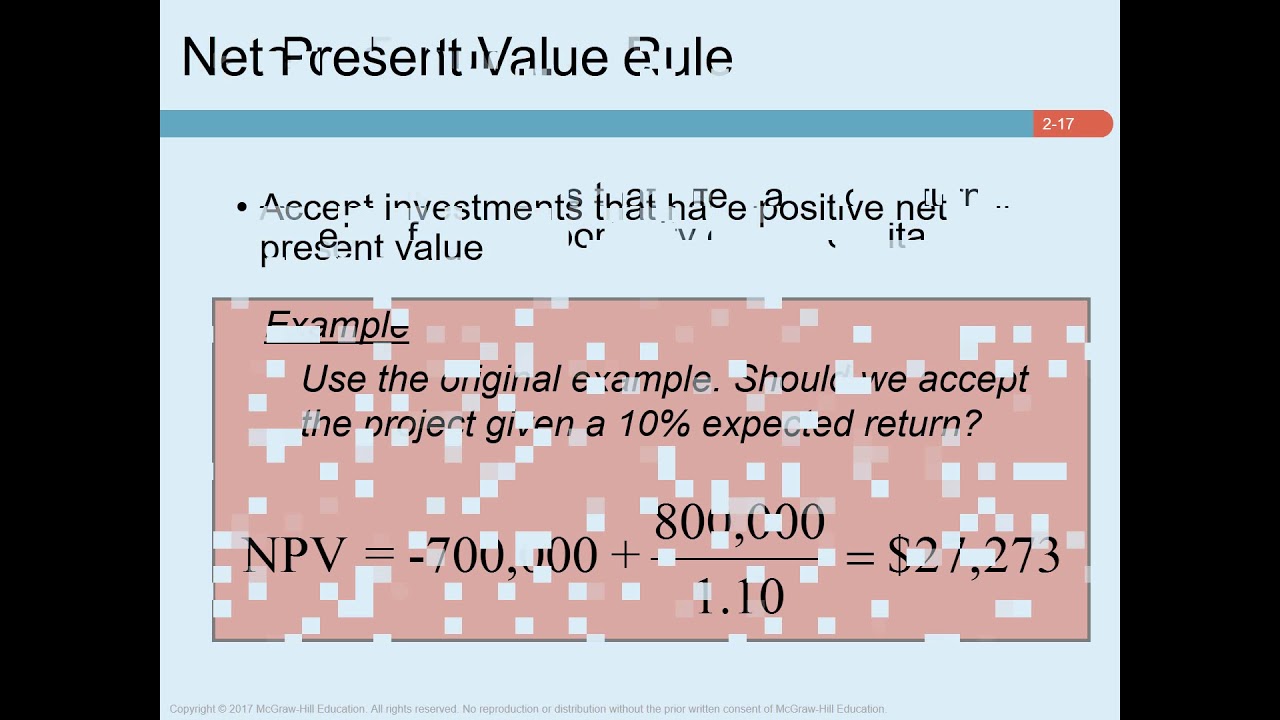
The NPV rule is straightforward: accept investments with a positive NPV. This indicates that the investment adds value to the company, as the present value of cash inflows surpasses the invested amount. For example, an investment with cash inflows discounted by 10%, resulting in an NPV of $27,000, would be accepted based on this rule.

Rate of Return and Investment Decisions

Another way to evaluate investments is through the rate of return, which is the profit as a proportion of the initial investment. If the rate of return exceeds the cost of capital (opportunity cost), the investment is considered worthwhile. In the case of the office building project, a 14.3% rate of return against a cost of capital of 10% or 12% would make the project acceptable, as it generates an excess return.

Capital Investment Decision Rules

Investors have two equivalent decision rules for capital investment: the NPV rule and the rate of return rule. Both aim to ensure that investments provide returns in excess of their opportunity costs.

Extending NPV to Multiple Cash Flows

Projects often involve multiple cash flows over several periods, not just a single inflow. To calculate the NPV for such projects, each cash flow must be discounted to its present value, summed up, and then compared to the initial investment. Positive NPV in such scenarios still indicates a project worthy of acceptance.

For instance, a project with an initial investment of $700,000 and cash flows of $30,000 in year one and $870,000 in year two, at a discount rate of 12%, would have an NPV of $20,334.44. As this is positive, the project would be accepted.

Final Thoughts

The relationship between risk and present value is at the heart of investment decisions. Investors must weigh the risk-adjusted returns of potential projects against the certainty of risk-free investments. By understanding and applying NPV and rate of return rules, investors can make informed decisions that maximize value while managing risk.

For a deeper understanding of these concepts, watch the full lecture video.